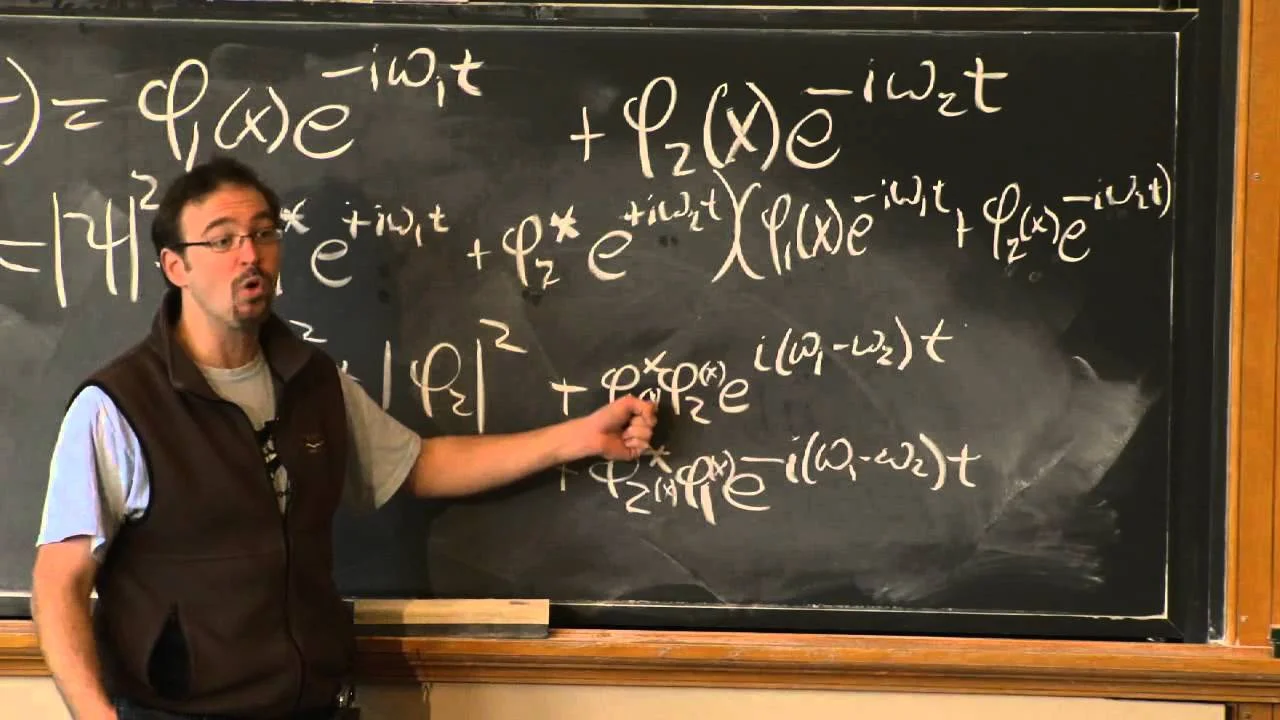The wavefunction \(\psi(L,t)\) is confined to a circle whenever the eigenvalues L of a particle are only nonzero on the points along a circle. When the wavefunction \(\psi(L,t)\) associated with a particle has non-zero values only on points along a circle of radius \(r\), the eigenvalues \(p\) (of the momentum operator \(\hat{P}\)) are quantized—they come in discrete multiples of \(n\frac{ℏ}{r}\) where \(n=1,2,…\) Since the eigenvalues for angular momentum are \(L=pr=nℏ\), it follows that angular momentum is also quantized.
Newton's second law describes how the classical state {\(\vec{p_i}, \vec{R_i}\)} of a classical system changes with time based on the initial position and configuration \(\vec{R_i}\), and also the initial momentum \(\vec{p_i}\). We'll see that Schrodinger's equation is the quantum analogue of Newton's second law and describes the time-evolution of a quantum state \(|\psi(t)⟩\) based on the following two initial conditions: the energy and initial state of the system.
In this section, we'll begin by seeing how Schrodinger's time-independent equation can be used to determine the wave function of a free particle. After that, we'll use Schrodinger's time-independent equation to solve for the allowed, quantized wave functions and allowed, energy eigenvalues of a "particle in a box"; this will be useful later on as a qualitative understanding of the quantized wave functions and energy eigenvalues of atoms.
In general, if a quantum system starts out in any arbitrary state, it will evolve with time according to Schrödinger's equation such that the probability \(P(L)\) changes with time. In this lesson, we'll prove that if a quantum system starts out in an energy eigenstate, then the probability \(P(L)\) of measuring any physical quantity will not change with time.