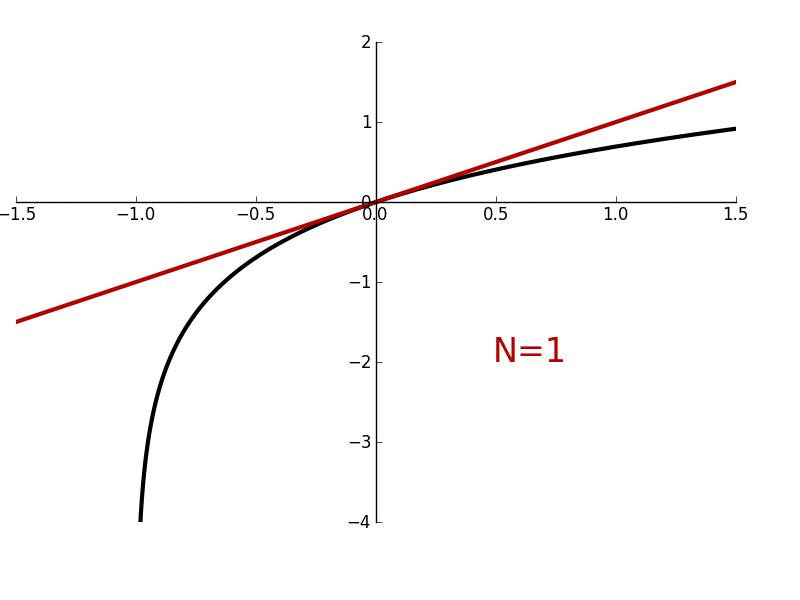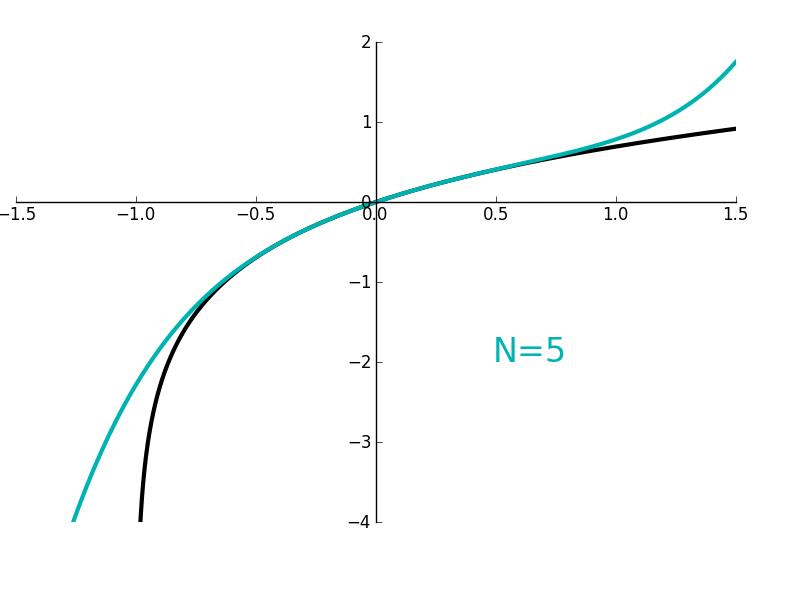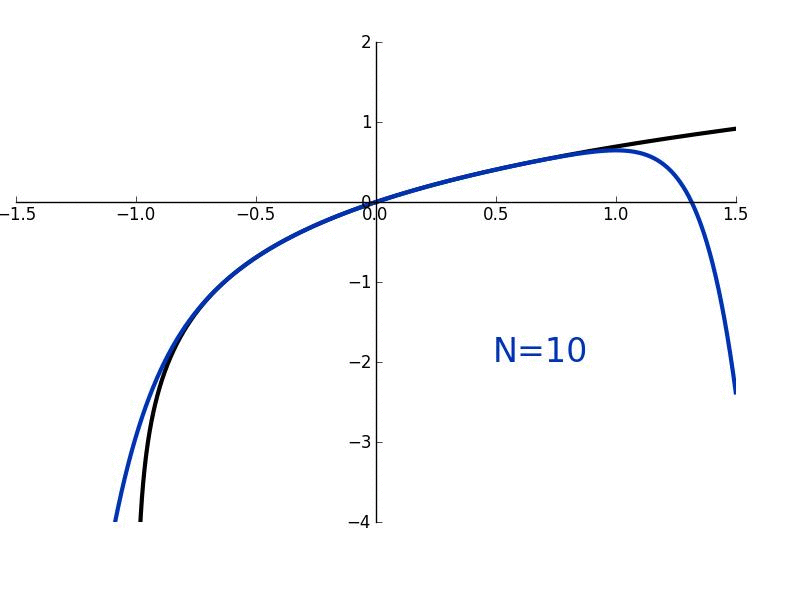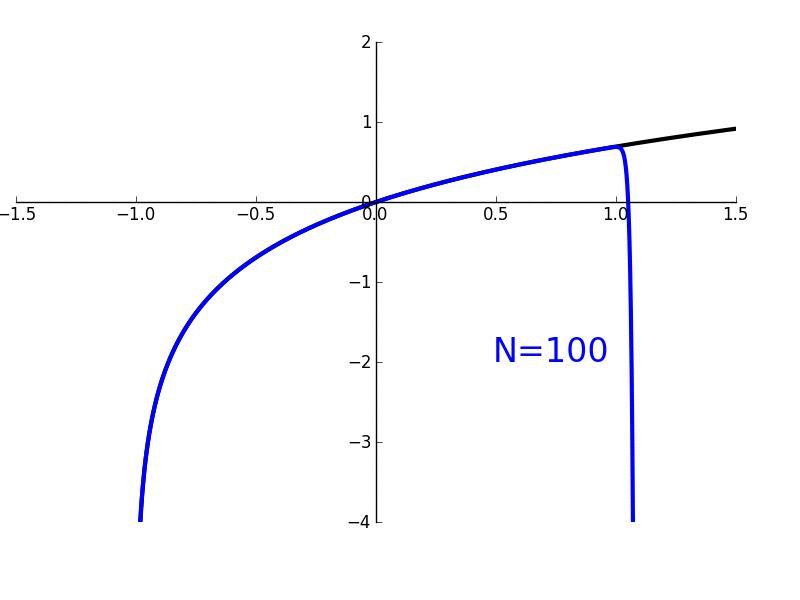In previous lessons, we learned that by taking the integral of some function \(f(x)\) we can find the area underneath that curve by summing the areas of infinitely many, infinitesimally skinny rectangles. In this lesson, we'll use the concept of a double integral to find the volume underneath any smooth and continuous surface \(f(x,y)\) by summing the volumes of infinitely many, infinitesimally skinny columns.
How to Produce Water and Oxygen on Mars
The lack of oxygen in Mars' atmosphere and running liquid water on its surface is very inconveniant for any humans living their since oxygen and liquid water are necessary for humans to survive. Fortunatelly, there is an abundance of frozen water on Mars' surface. In this lesson, we'll discuss various techniques which can be used to extract all of this water. Once the water is obtained, by performing electrolysis on the water we can distill all of the oxygen from that water we need.
Colonizing and Terraforming Venus
The first serious proposal in scientific literature on terraforming other worlds in the universe was about terraforming Venus. The planetary scientist Carl Sagan imagined seeding the Venusian skies with photosynthetic microbes capable of converting Venus's \(C0_2\)-rich atmosphere into oxygen. Other proposals involve assembling a vast system of orbital mirrors capable of blocking the Sun's light and cooling Venus until this hot and hellish world became very frigid and rained \(C0_2\) from its atmosphere. The solleta would also be capable of simulating an Earth day/night cycle. To create oceans and an active hydrosphere on Venus, we could hurl scores of icy asteroids from the Kuiper belt to Venus and, upon impacting the Venusian atmosphere, would rapidly disintegrate releasing enormous quantities of water vapor into the atmosphere which subsequently condense to form the first seas on Venus. Or perhaps Saturn's moon Enceladus—containing a colossal subsurface ocean dwarfing that of the Earth's—could be sacrificed towards the end of creating the first seas on Venus. But even if humans never terraform this hellish world, they could still live their—partially at least—by deploying thousands of blimps into the Venusian skies capable of supporting a long-term, human presence of perhaps over a million people. Venusian sky cities. But eventually, after many millennia of terraforming Venus, a rich ecosystem of life—including us—could live on Venus's surface.
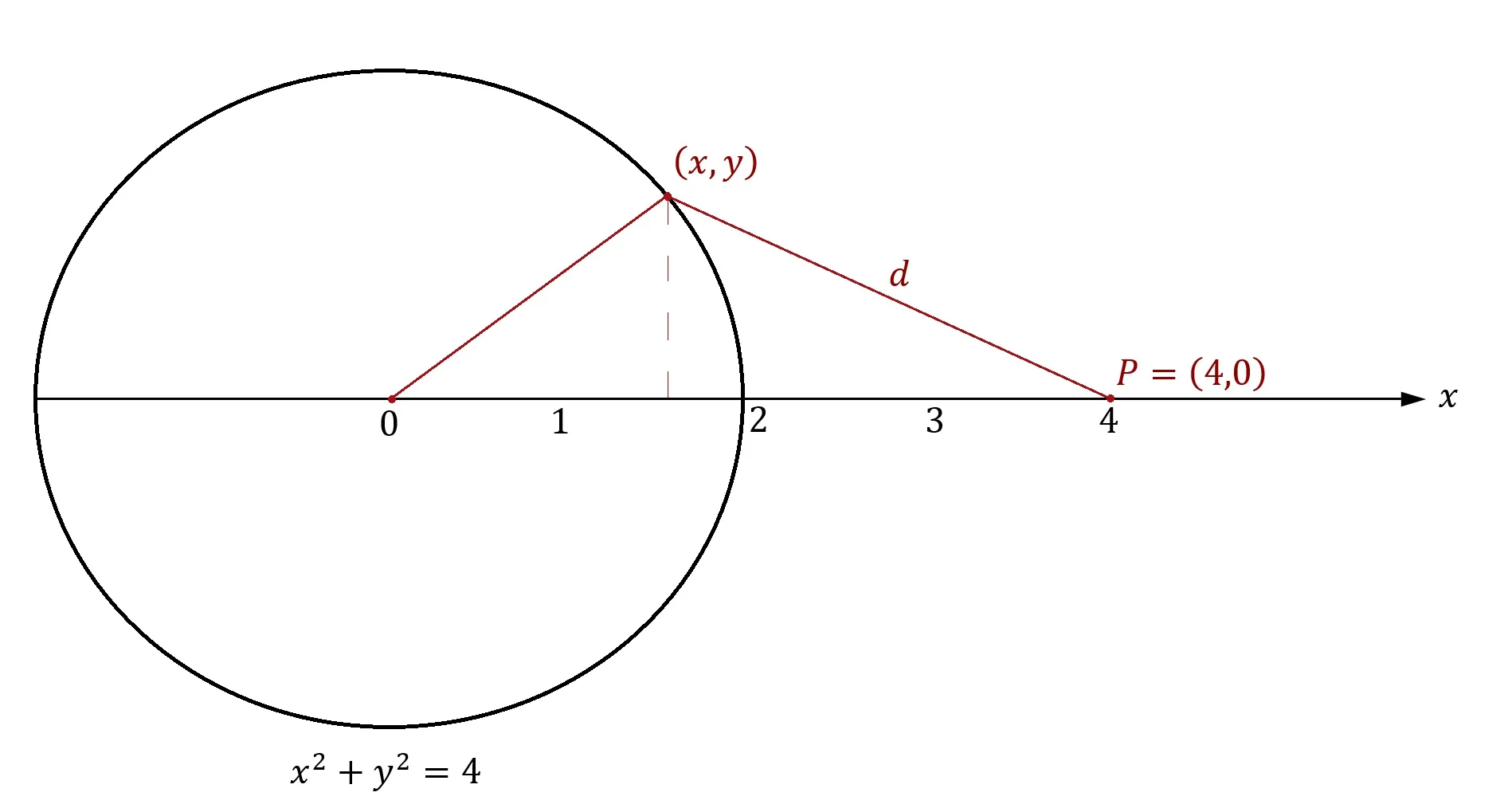
Optimization Problem
If \((x,y)\) represents any point on the circle, if \(P\) is a point fixed at the coordinate point \((4,0)\), and if \(d\) represents the distance between those two points then, by using only calculus, we can find the point \((x,y)\) on the circle associated with the minimum distance \(d\).
Maximizing the Area of a Rectangle
Given that the perimeter \(2x+2y\) of any arbitrary rectangle must be constant, we can use calculus to find that particular rectangle with the greatest area. The solution to this problem has practical applications. For example, suppose that someone had only 30 meters of fencing to enclose their backyard and they wanted to know what fencing layout would maximize the size and total area of their backyard. Using calculus, we can answer such questions.
Finding the Minima and Maxima of a Function
Calculus—specifically, derivatives—can be used to find the values of \(x\) at which the function \(f(x)\) is at either a minimum value or a maximum value. For example, suppose that we let \(x\) denote the horizontal distance away from the beginning of a hiking trail near a mountain and we let \(f(x)\) denote the altitude of the mountainous terrain at each \(x\) value. \(f(x)\) reaches a minimum value when the function "flattens out"—that is, when \(f'(x)\) becomes equal to zero. These particular values of \(x\) are associated with the bottom and top of the mountain. The condition that \(f'(x)=0\) only tells us that \(f(x)\) is at either a minimum or a maximum. To determine whether or not \(f(x)\) is at a minimum or a maximum, we must use the concept of the second derivative. This will be the topic of discussion in this lesson.
Colonizing the Kuiper Belt and Oort Cloud
The icy asteroids and comets in the Kuiper belt will one day (perhaps during the 22nd century) be hurled towards Mars’ atmosphere where they’ll disintegrate and release nitrogen into the atmosphere—a crucial step in the project of terraforming Mars. In the future, asteroids will perhaps be used as spaceships powered by nuclear reactors. The fuel for these nuclear reactors—deuterium and helium-3—could be harvested from the asteroids and the atmospheres of gas giants, respectively. As the famous physicist Freeman Dyson once noted, since the Oort Cloud contains all the ingredients necessary to support life, this realm of a trillion or more comets will likely be a way point for a long voyage to the Alpha Centuari star system.
Why Colonize the Universe?
In Carl Sagan's book Pale Blue Dot, he argued that humans evolved a love for exploration as an essential part of our survival as a species. It was this evolutionary trait which compelled our hunter-gather ancestors to leave their home—Africa—when times were getting rough and to meander across the planet. As planetary catastrophes become increasingly likely as time rolls by, Sagan argues that this same "survival strategy" will perhaps compel humanity to colonize the solar system, and beyond. Even the universe itself will one day become too dangerous for humans to live in and we’ll need to voyage to another universe to survive.
Calculating the Arc Length of a Curve
In this lesson, we'll use the concept of a definite integral to calculate the arc length of a curve.
The Kardeshev Scale
The Kardeshev scale ranks how advanced a technological civilization is based on its power consumption. A Type I civilization is a civilization which has harnessed all of their planet's renewable energy sources and who can control the natural forces of their planet such as the weather and volcanoes; a Type II civilization has harnessed the total power output of their home-star and routinely move and dissemble stars; a Type III civilization is one which has spread across the entire galaxy and harnessed the total power output of their galaxy; a Type IV civilization is one with faster-than-light (FTL) speed spacecraft and that has harnessed the total power output of all the galaxies in their universe; Type V civilizations are like gods which have colonized other universes and can spontaneously create other universes at will.
Solving Problems using Line Integrals
In the previous lesson, we defined the concept of a line integral and derived a formula for calculating them. We learned that line integrals give the volume between a surface \(f(x,y)\) and a curve \(C\). In this lesson, we'll learn about some of the applications of line integral for finding the volumes of solids and calculating work. In particular, we'll use the concept of line integrals to calculate the volume of a cylinder, the work done by a proton on another proton moving in the presence of its electric field, and the work done by gravity on a swinging pendulum.
Introduction to Line Integrals
Line integrals are used to find the area between a surface \(f(x,y)\) and any arbitrary curve. In this lesson, we'll define the idea of line integral and derive a formula for calculating them.
Maclaurin/Taylor Polynomials and Series
In this lesson, we'll derive Maclaurin/Taylor polynomials which are used to "approximate" arbitrary functions which are smooth and continuous. More generally, they are used to give a local approximation of such functions. We'll also derive Maclaurin/Taylor series where the approximation becomes exact.
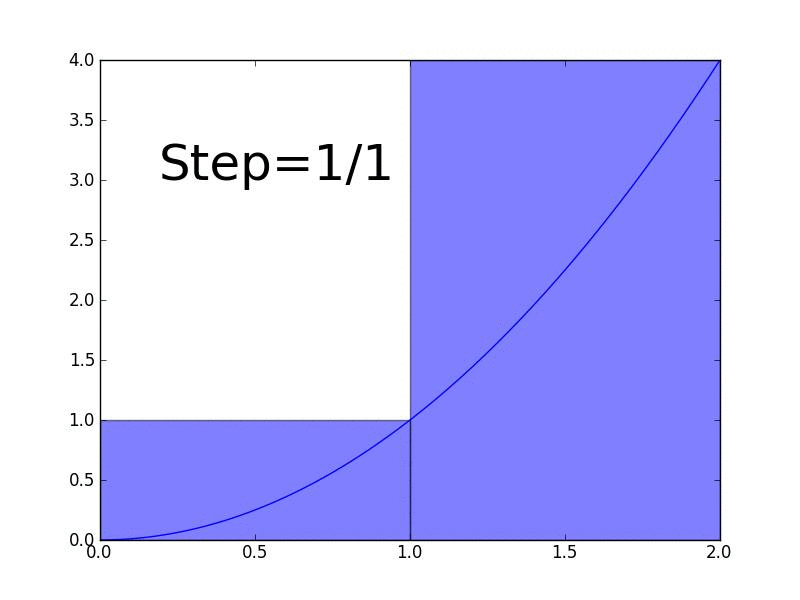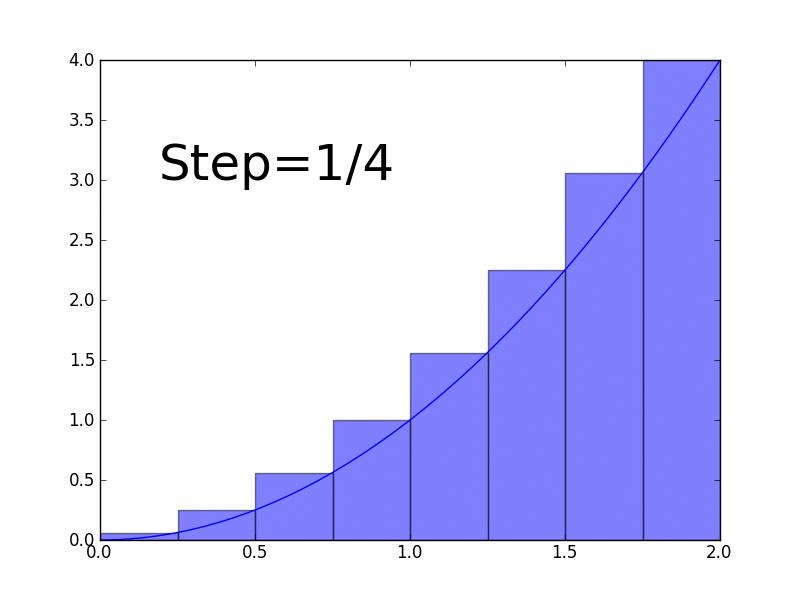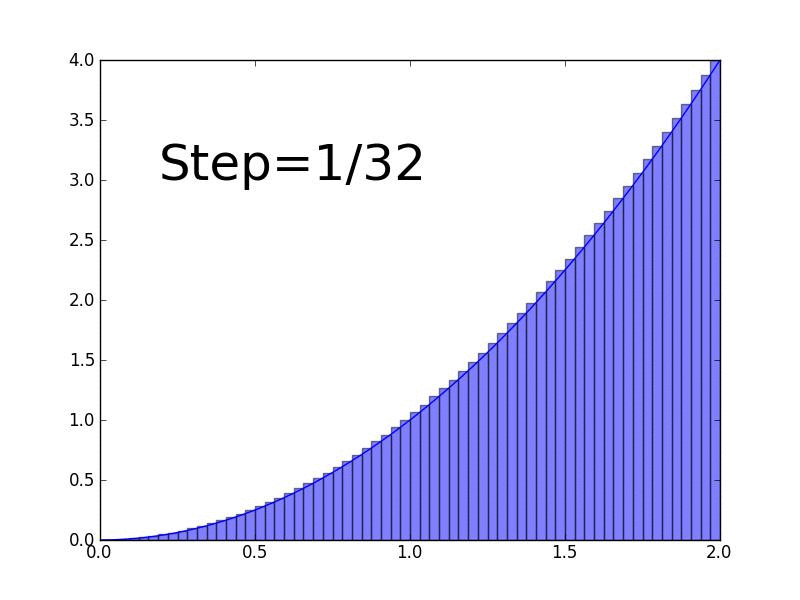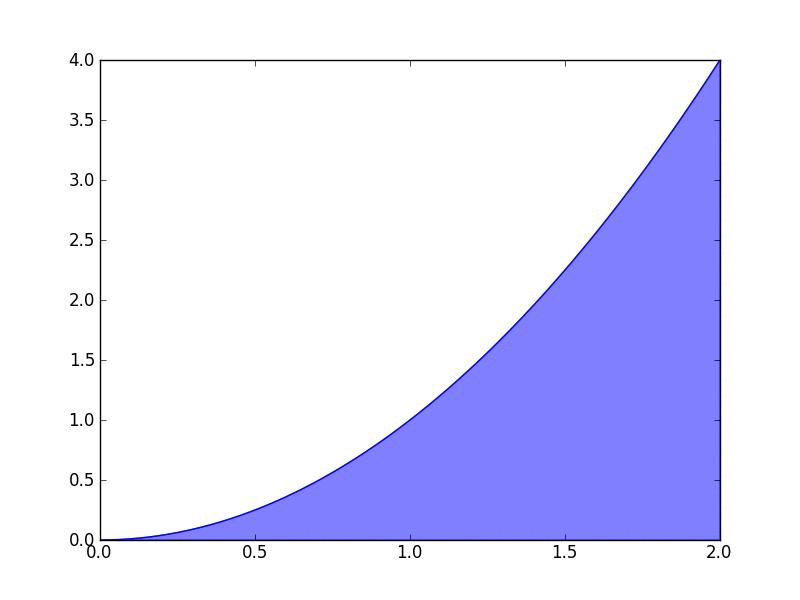
Introduction to Integrals
In this lesson, we define an integral as the Riemann sum as the number of rectangles approaches infinity.
Alcubierre Warp Drive
A spaceship using an Alcubierre warp drive would involve assembling a ring of negative energy around the spaceship which would distort spacetime in this particular way: the spaceship sits in a "bubble" of flat, Minkowski spacetime which is "pushed" by expanding space behind it and "pulled" by contracting space infront of it. The spaceship does not move through space, but rather space itself moves and carries along the spaceship for the ride. Since general relativity places no limit on how fast space can move, the space can "carry" the warp bubble and spaceship away at faster than light (FTL) speeds.
Terraforming and Colonizing Mars
Terraforming a world just means to make it more Earth-like. In this article, we'll discuss various techniques which have been proposed by scientists and engineers that would make Mars more like our home planet. We shall also discuss a potential scheme of future events which might occur as humans terraform and colonize Mars.
Introduction to Special Relativity
When Einstein was first working on developing his special theory of relativity, he was studying both Newtonian mechanics and Maxwell's equations: the two pillars of modern physics of his time. He noticed that these two theories contradicted one another in a very deep way. One of these pillars must fall. Newtonian mechanics implies the Galilean transformation equations—something which we are all already familiar with. These equations are consonant with our everyday intuitions: they tell us that velocities add. This makes sense: If I watch someone standing on top of semi throw a baseball at 5 m/s (in the same direction the truck is moving) and the truck wizzes by me at 30 m/s, then I'll see the baseball travel away from me at 35 m/s. But Maxwell's equations predict something very strange. These equations predict that a light beam will move away from you at the same speed whether your standing still or, as in another one of Einstein's thought experiments, "chasing" the light beam at 99% the speed of light. The reconciliation between Newtonian mechanics and Maxwell's equations is called the special theory of relativity. When we modify Newtonian mechanics to allow for the constancy of light speed for all observers, we get some very strange, bizarre, but also very profound consequences.
Time Dilation
Time dilation is one of the many bizarre consequences of the speed of light being the same for everybody. For any observers in inertial frames of reference moving away from one another at a relative velocity which is close to that of the speed of light, they will see each others clocks run more slowly. In this section, we'll explore one of Einstein's original thought experiment: a train with a light clock moving past an observer standing idly at the train station. We'll see that the constancy of light speed with respect to all observers implies that time must slow down when watching events unfold in one frame of reference from another frame of reference if those two frames of reference are moving relative to each other. We'll start off by showing this for light beams bouncing off of two mirrors, but at the end we'll realize that this same argument applies to photons emitted between two atoms. The latter results in all physical clocks, chemical or biological, running more slowly.
Time-Independant Schrodinger Equation: Free Particle and Particle in One-Dimensional Box
In this section, we'll begin by seeing how Schrodinger's time-independent equation can be used to determine the wave function of a free particle. After that, we'll use Schrodinger's time-independent equation to solve for the allowed, quantized wave functions and allowed, energy eigenvalues of a "particle in a box"; this will be useful later on as a qualitative understanding of the quantized wave functions and energy eigenvalues of atoms.
Light Fidelity (Li-Fi): Ultra-Fast, Wireless Communications System
Li-Fi was invented in 2011 by professor Harald Haas and is a form of wireless communications technology which would allow us to transmit information and data at least 100 times faster than Wi-Fi. Even more significantly, Li-Fi is essential—and in fact, it is necessary—for us to transition to a Third Industrial Revolution (TIR) infrastructure where everything in the environment—from buildings, roads, and walkways—becomes "cognified."